Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us! The derivative of sin x is cos x, The derivative of cos x is −sin x (note the negative sign!) and The derivative of tan x is sec 2x Now, if u = f(x) is a function of x, then by using the chain rule, we have d ( sin u) d x = cos u d u d x The derivative of sec x tan x In simple language, keep the initial term as it is and distinguish the second term, then distinguish the first term and keep the next term since it is or viceversa What is the derivative of SEC 2x?
What Is The Integration Of Tanx Tan2x Tan3x Dx Quora
Derivative of tan 2x 3
Derivative of tan 2x 3-An older video where Sal finds the derivative of tan (x) using the quotient rule Created by Sal Khan Trigonometric functions differentiation Derivatives of tan (x) and cot (x) Derivatives of sec (x) and csc (x) Practice Derivatives of tan (x), cot (x), sec (x), and csc (x) Worked example Derivative of sec (3π/2x) using the chain ruleAt a point , the derivative is defined to be This limit is not guaranteed to exist, but if it does, is said to be differentiable at Geometrically speaking, is the slope of the tangent line of at As an example, if , then and then we can compute The derivative is a




Derivatives Of Tan X And Cot X Video Khan Academy
Advanced Math questions and answers 1 *P (x) = Find derivative of the following function fx) = tann (2x FU)= cos (5), X 7x)sine) f (x)tan/3 xv1in (2x) Gear √ 76) 1 सन xvx 07) = E * pW) Tin telin) 1 * (1 In22x) 1 (1 V3 27 (1 3x) 3 2X (13) H Example 22 Find the derivative of tan (2x 3) Let y = tan (2x 3) We need to find derivative of y, ie 𝑑𝑦/𝑑𝑥 = (𝑑 tan〖(2𝑥3)〗)/𝑑𝑥Solution for 5 Find the derivative of the following 3x5 а у3 b y = In (sin(3x5)) c y= e2x arc tan (2x 3) x1
The Derivative tells us the slope of a function at any point There are rules we can follow to find many derivatives For example The slope of a constant value (like 3) is always 0; d/dx tan^2(3x) = 6sec^2(3x)tan(3x) In order to differentiate this function, we have to apply the chain rule twice d/dx tan(f(x))= sec^2(f(x)) f'(x) d/dx tan(x)^n = ntan(x)^(n1)sec^2x So, applying these two rules, we get d/dx tan^2(3x) = 2tan(3x)sec^2(3x)(3)=6sec^2(3x)tan(3x)To apply the Chain Rule, set u u as 2 x − x 3 2 x x 3 The derivative of tan ( u) tan ( u) with respect to u u is sec 2 ( u) sec 2 ( u) Replace all occurrences of u u with 2 x − x 3 2 x x 3 Differentiate Tap for more steps By the Sum Rule, the derivative of 2 x − x 3 2 x x 3 with respect to x x is d d x 2 x d d x − x
Derivative calculator This calculator evaluates derivatives using analytical differentiation It will also find local minimum and maximum, of the given function The calculator will try to simplify result as much as possibleDerivative of tan^2 (x) \square! As stated previously, the derivative of a function is a measure of how sensitive the output of a function is to changes in its input The derivative of ƒ(x) measures the rate of change of the output of ƒ(x) with respect to changes in x Imagine the simple case where we have some linear equation y=2x3 Further, lets pick two sets of x y



Differentiate The Following From First Principles I Tan 2 X Ii Tan 2x 1 Sarthaks Econnect Largest Online Education Community
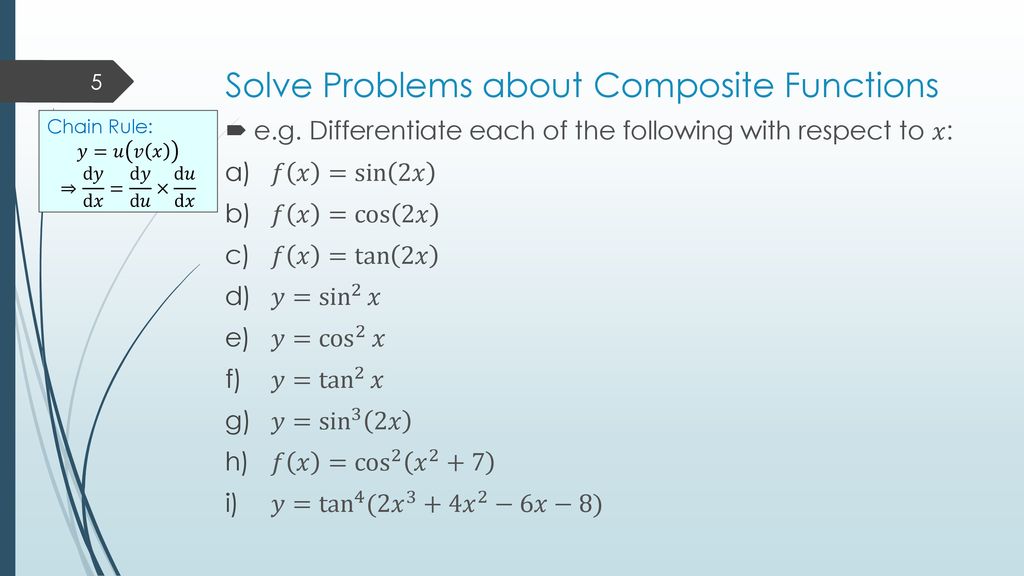



Differentiation With Trig Outcomes Ppt Download
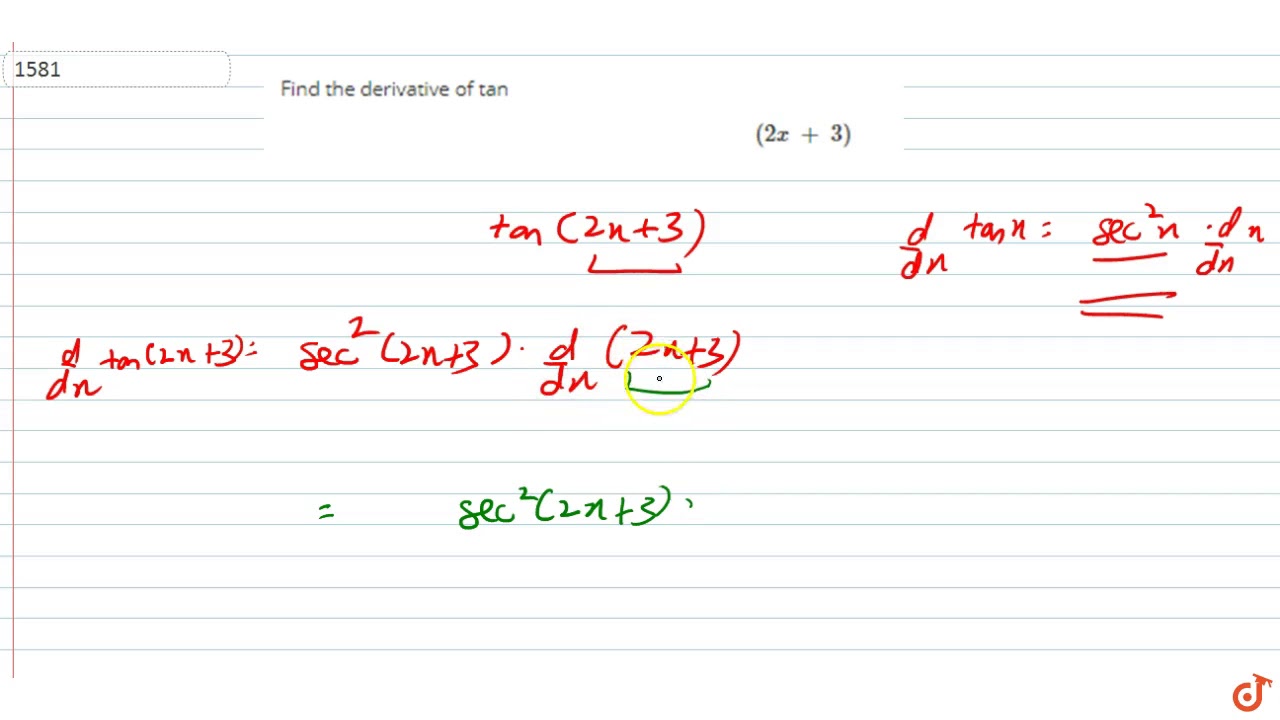
Ex 53, 10 Find 𝑑𝑦/𝑑𝑥 in, 𝑦 = tan–1 ((3𝑥− 𝑥^3)/( 1− 3𝑥2 )) , − 1/√3 < 𝑥 < 1/√3 𝑦 = tan–1 ((3𝑥− 𝑥^3)/( 1− 3𝑥2 find the derivative of tanx by first principle Mathematics TopperLearningcom iyyne Topperlearning CBSE Class 11science Ask The Expert AnsweredFind the derivative of (tan 2x plus 3) out of the following options 1 sec(2x 3) 2 secx 3 logsec(2x 3) Maths Continuity and Differentiability



05 Derivative Of Tangent Function Tan2x And Tanx 2 Youtube




Solved 1 Find The Derivative Of Each Function A E E 7 Chegg Com
Click here👆to get an answer to your question ️ Derivative of tan ^1 ( x√(1 x^2) ) with respect to sin ^1 ( 3x 4x^3 ) isThe derivative of sec2 (x) is 2sectwo (x) tan (x) The chain rule says the derivative of f (g (x)) is equivalent toDerivative examples Example #1 f (x) = x 3 5x 2 x8 f ' (x) = 3x 2 2⋅5x10 = 3x 2 10x1 Example #2 f (x) = sin(3x 2) When applying the chain rule f ' (x) = cos(3x 2) ⋅ 3x 2' = cos(3x 2) ⋅ 6x Second derivative test When the first derivative of a function is zero at point x 0 f '(x 0) = 0 Then the second derivative at point x 0, f''(x 0), can indicate the type of that point




Derivatives Of Trigonometric Functions




Derivative Of Tan 2x 3
The slope of a line like 2x is 2, or 3x is 3 etc;The derivative of tan 2x is 2 sec 2 (2x) (ie) d/dx tan 2x = 2 sec 2 (2x) Explanation We know that the derivative of tan x is sec 2 x (ie) d/dx (tan x) = sec 2 x According to the chain rule,Derivative Proof of tan(x) Derivative proof of tan(x) We can prove this derivative by using the derivatives of sin and cos, as well as quotient rule Write tangent in terms of sine and cosine Take the derivative of both sides Use Quotient Rule Simplify Use the Pythagorean identity for sine and cosine and simplify Derivative




Find The Derivative Of Tan 2x 3 Youtube
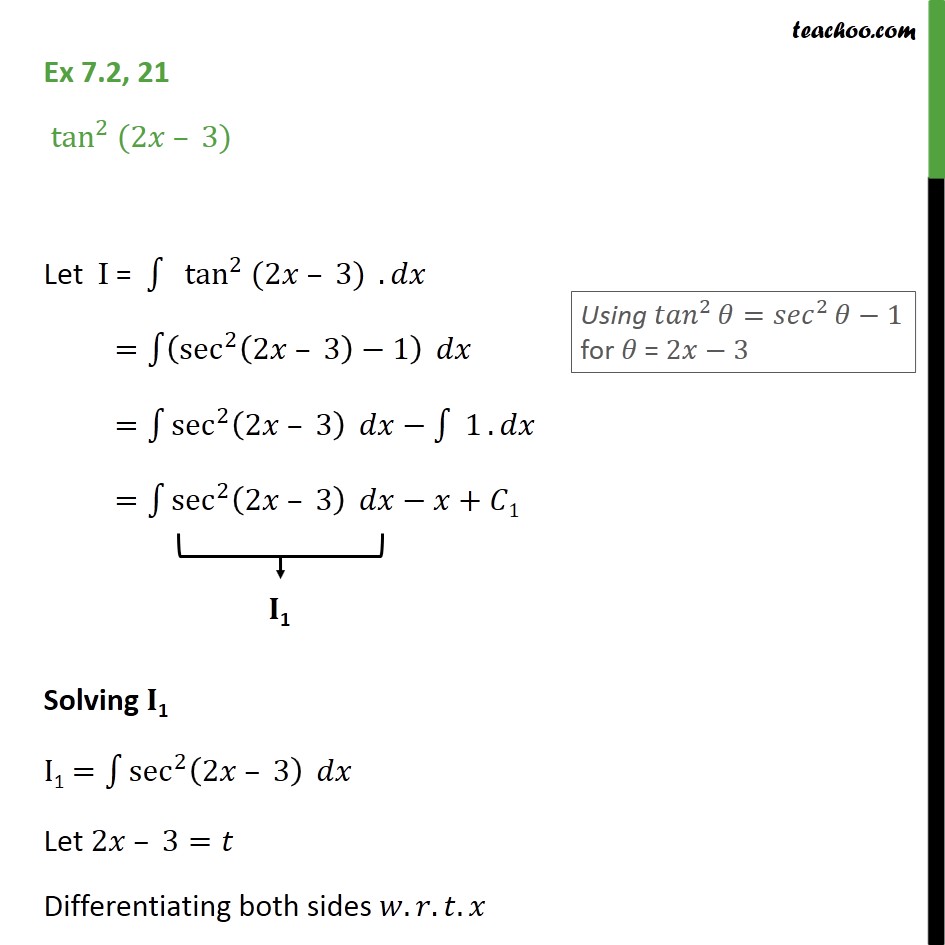



Ex 7 2 21 Integrate Tan2 2x 3 Class 12 Cbse Ex 7 2
The tangent line is the best linear approximation of the function near that input value For this reason, the derivative is often described as the "instantaneous rate of change", the ratio of the instantaneous change in the dependent variable to that of the independent variable The derivative of tan x The derivative of tan x is sec 2 x Finally, just a note on syntax and notation tan (3x) is sometimes written in the forms below (with the derivative as per the calculation above) Just be aware that not all of the forms below are mathematically correct tan3x Derivative of tan3x = 3sec 2 (3x) tan 3 x Derivative of tan 3 x = 3sec 2 (3x) tan 3xY = tan^3(x) , find the derivative




Solved Differentiate The Following Function With Respect To Chegg Com




13 10 The Derivative Of Tan 2x With Respect To 1 2 B T1 X
Derivative of the function will be computed and displayed on the screen Click on 'Show a step by step solution' if you would like to see the differentiation steps Click on 'Draw graph' to display graphs of the function and its derivative Common errors while using derivative calculator About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us Creators The Second Derivative Of tan^2x To calculate the second derivative of a function, differentiate the first derivative From above, we found that the first derivative of tan^2x = 2tan(x)sec 2 (x) So to find the second derivative of tan^2x, we need to differentiate 2tan(x)sec 2 (x) We can use the product and chain rules, and then simplify to find the derivative of
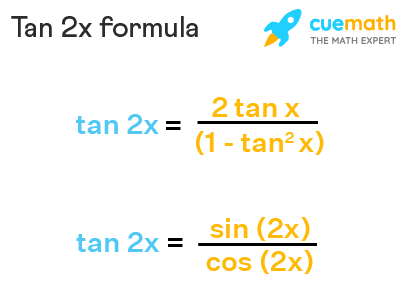



Tan 2x Formula What Is Tan 2x Formula Examples




Derivative Of Inverse Tangent Video Khan Academy
Find the derivative of following functions wrt x tan (2x 3) Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to their queriesInteractive graphs/plots help visualize and better understand the functionsThe derivative of any function y = f(x) of a variable x is a measure of the rate at which the value y changes with respect to the change of x Answer The derivative of y = tan 2 x is dy/dx = 2 sec 2



1



Chapter 3 Differentiation 1 3 1 The Derivative
Share It On Facebook Twitter Email 1 Answer 1 vote answered by Reyansh (191k points) selected by faiz Best answer Let f(x) = tan(2x 3)Derivative of \(tanx = sec^2x \) What Is The Derivative Of tan(x)?Calculus questions and answers 1 Find the derivative of f (x) = tan (In x) 532 2 Find the derivative of f (x) = log2 (tan x) In (1) 1 3 Find the derivative of y = ln (In (In x)) 4 Find the derivative of y=logs (e* cos (2x)) Question 1 Find the derivative of f (x) = tan (In x) 532 2 Find the derivative of f (x) = log2 (tan



Derivative Of Tangent X Sec X Tan X Longer Free Tutorial Get Education Bee




Derivative Of Tan X Proof In Easy Steps Calculus How To
The derivative of tan x with respect to x is denoted by d/dx (tan x) (or) (tan x)' and its value is equal to sec 2 x Tan x is differentiable in its domain To prove the differentiation of tan x to be sec 2 x, we use the existing trigonometric identities and existing rules of differentiation We can prove this in the following ways Proof by first principle\( \frac{d}{dx} {tanx} = \frac{d}{dx} \frac{sinx}{cosx}\) we know that \( tanx =\frac{sinx}{cosxAnswer to Find the derivative of g(x) = x^3 tan(2x) By signing up, you'll get thousands of stepbystep solutions to your homework questions You




The Derivative Of Tan X Is Sec 2 X Why Mathematics Stack Exchange




Find The Derivative Of Tan 2x 3 Scholr
The Derivative of the Tangent Function Find the derivative of f (x) = tan x f (x) = tan x Solution Start by expressing tan x tan x as the quotient of sin x sin x and cos x cos x f (x) = tan x = sin x cos x f (x) = tan x = sin x cos x Now apply the quotient rule to obtain f y − 1 = −2 (xIf one of the zeros of the quadratic polynomial (k1) x2 kx 1 is 3, then find the value of k Find the prime factorisation of 980 What are the factors of 216 and 215?To apply the Chain Rule, set u u as tan ( x) tan ( x) Differentiate using the Power Rule which states that d d u u n d d u u n is n u n − 1 n u n 1 where n = 3 n = 3 Replace all occurrences of u u with tan ( x) tan ( x) The derivative of tan(x) tan ( x) with respect to x x is sec2(x) sec 2 ( x) Reorder the factors of 3tan2(x)sec2




Derivatives Of Tan X And Cot X Video Khan Academy
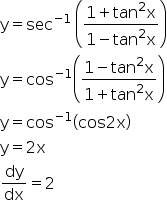



Differentiate Wrt X Sec 1 1 Tan2x 1 Tan2x Mathematics Topperlearning Com Nti7rxx
Derivative of tan (2x)^3 \square!In the case of antiderivatives, the entire procedure is repeated with each function's derivative, since antiderivatives are allowed to differ by a constant The interactive function graphs are computed in the browser and displayed within a canvas element (HTML5) For each function to be graphed, the calculator creates a JavaScript functionAnd so on Here are useful rules to help you work out the derivatives of many functions (with examples below)Note the little mark ' means derivative of, and
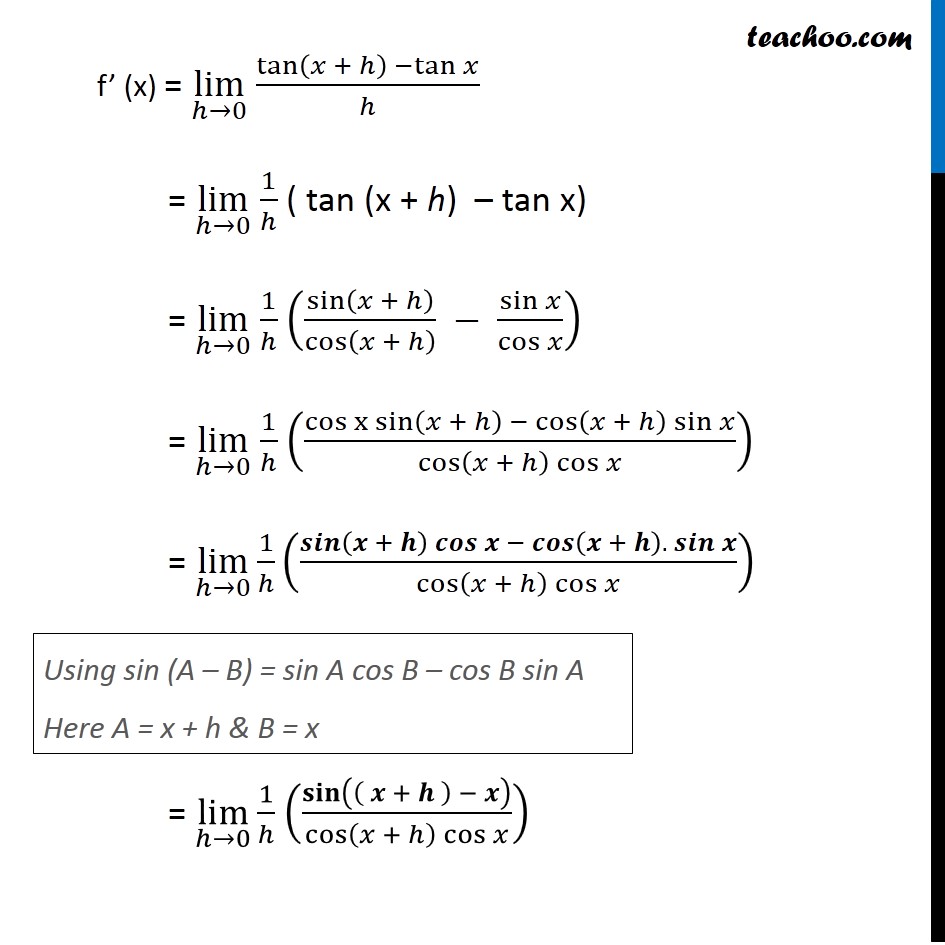



Prove That Derivative Of Tan X Is Sec 2 X By First Principle



What Is The Integration Of Tanx Tan2x Tan3x Dx Quora
Eg1 Write sinxcosxtanx as sin(x)cos(x)tan(x) 2 Write secx*tanx as sec(x)*tan(x) 3 Write tanx/sinx as tan(x)/sin(x) 4 Use inv to specify inverse and ln to specify natural log respectively Eg1 Write sin1 x as asin(x) 2 Write ln x as ln(x) 5 Sample Inputs for Practice Eg1 Write (10x2)(x 2) as 10*x2x^2 2 Write cos(x 3) as cos Find the derivative of the function tan (2x 3) from the definition (first principles) class12;Answer (1 of 4) Let, u = (tanx)^2 and v = (cosx)^2 Now differentiating u and v with respect to x Then du/dx = 2 tanx (secx)^2 And dv/dx = 2 cosx(sinx) Now (du/dx




Ex 7 2 21 Integrate Tan2 2x 3 Class 12 Cbse Ex 7 2




Tan 2x Formula What Is Tan 2x Formula Examples
Derivative of tan(2x)^3 Simple step by step solution, to learn Simple, and easy to understand, so don`t hesitate to use it as a solution of your homework Below you can find the full step by step solution for you problem We hope it will be very helpful for you and it will help you to understand the solving processAnswer (1 of 4) Let f(x)=\tan^3 (x) We can express f(x) as a composite function Let g(x)=x^3 and h(x)= \tan (x) f(x)=g(h(x)) Using the chain rule, we know that f'(xGet stepbystep solutions from expert tutors as fast as 1530 minutes



1



Differentiate The Sec 1 1 Tan 2x 1 Tan 2x W R T X Sarthaks Econnect Largest Online Education Community
The Derivative of y = tan2x is dy / dx = 2 sec^2x tan x 2 x? {eq}\displaystyle \sec^{2}(x) {/eq} is the derivative of tan(x) for x One can find the derivative of tan(x) using the definition of tan(x) in terms of 3 Derivatives of the Inverse Trigonometric Functions by M Bourne Recall from when we first met inverse trigonometric functions " sin1 x" means "find the angle whose sine equals x" Example 1 If x = sin1 025 then by using the calculator, x = 15° We have found the angle whose sine is 025




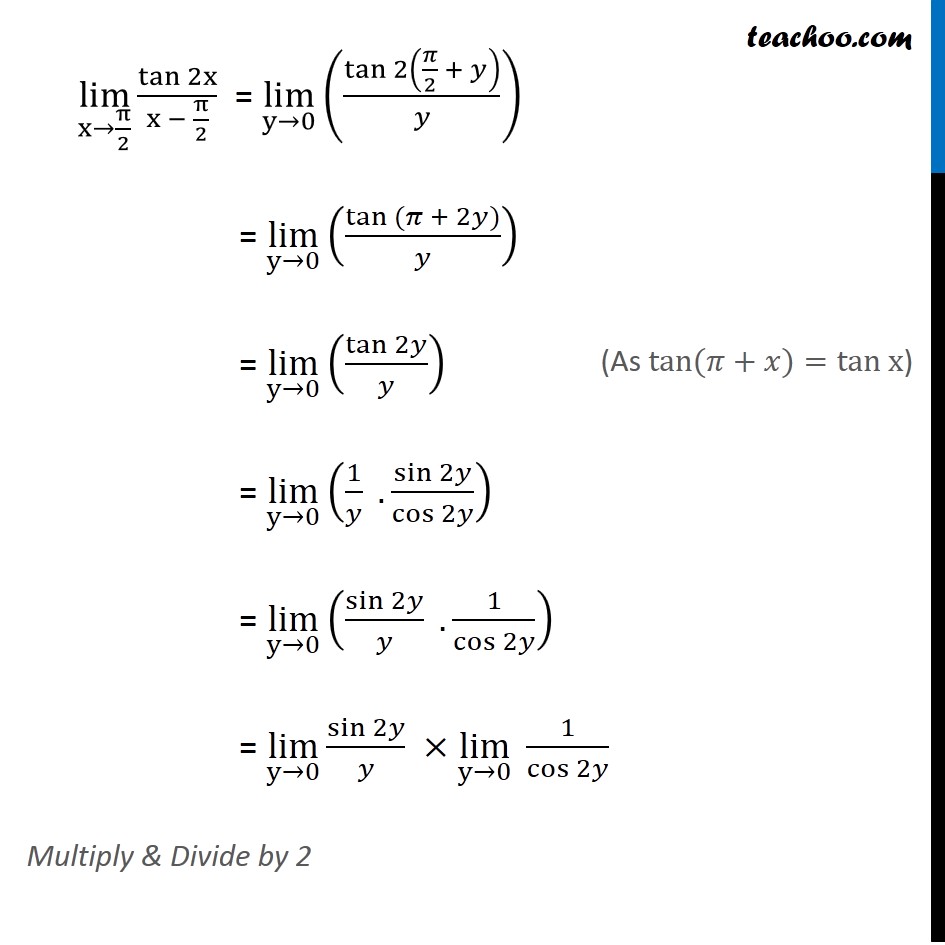
Ex 13 1 22 Lim X Pi 2 Tan 2x X Pi 2 Chapter 13 Class 11




How Do You Differentiate Y Sin X 6 Tan X 2 X 2 2 2 Socratic
The Derivative Calculator supports computing first, second, , fifth derivatives as well as differentiating functions with many variables (partial derivatives), implicit differentiation and calculating roots/zeros You can also check your answers! I have seen the derivative of $\tan^{1}(x)$ set equal to both $\sec^{2}(x)$ and $\frac{1}{1x^2}$ I can't find corroborating sources for either claim, soGraph of tan x and its Derivative The graphs of \( \tan(x) \) and its derivative are shown below Derivative of the Composite Function tan (u(x)) We now have a composite function which is a function (tan) of another function (u)




Derivative Of Tan 2x 3 Is A 2sec 2x 3 B Sec 2x 3 C Sec 2x 3 D None Of These Snapsolve
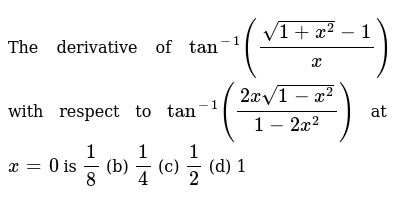



The Derivative Of Tan 1 Sqrt 1 X 2 1 X With Respect To Tan 1 2xsqrt 1 X 2 1 2x 2 At X 0 Is 1 8 B 1 4 C 1 2 D 1




The Derivative Of Tan 2x Derivativeit
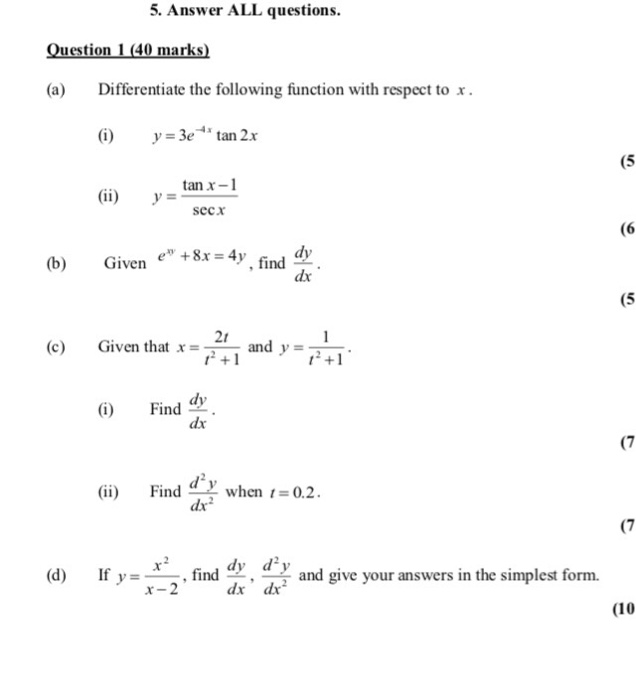



Solved A Differentiate The Following Function With Respect Chegg Com




How To Take The Derivative Of Tan X Video Lesson Transcript Study Com




Tan2x ただの悪魔の画像
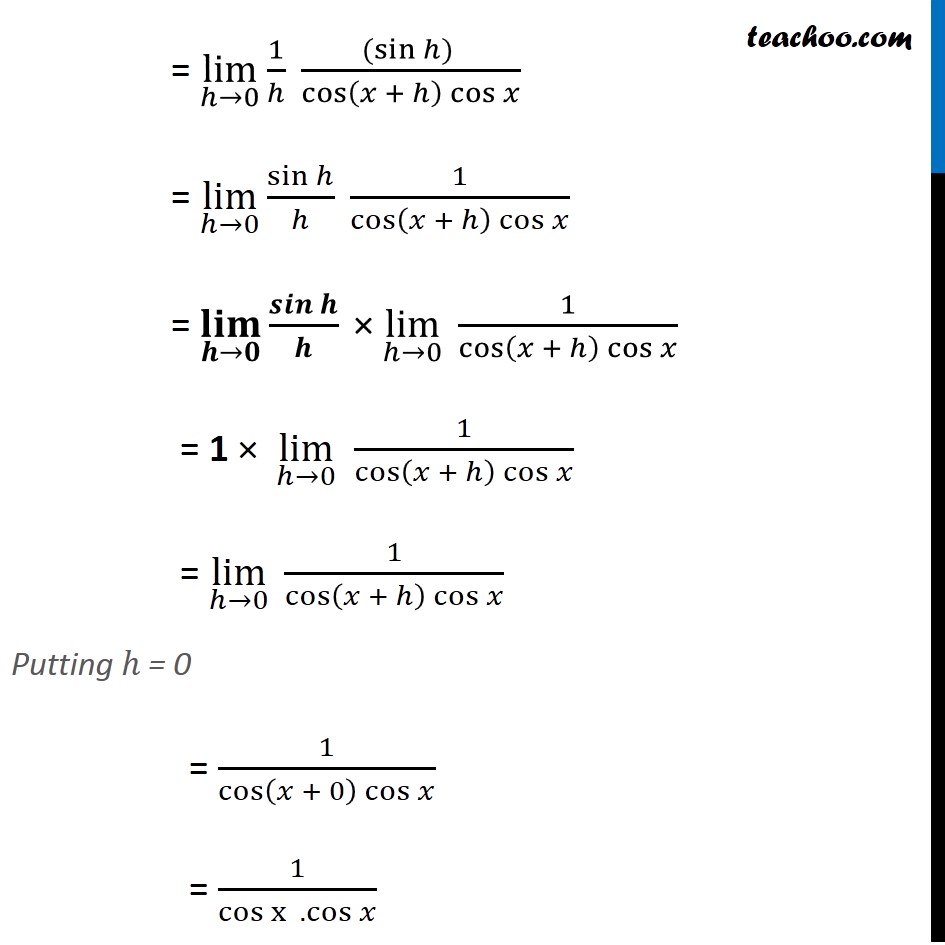



Prove That Derivative Of Tan X Is Sec 2 X By First Principle



Derivative Of Tan X



1




Second Derivative Of Tan 2x Youtube
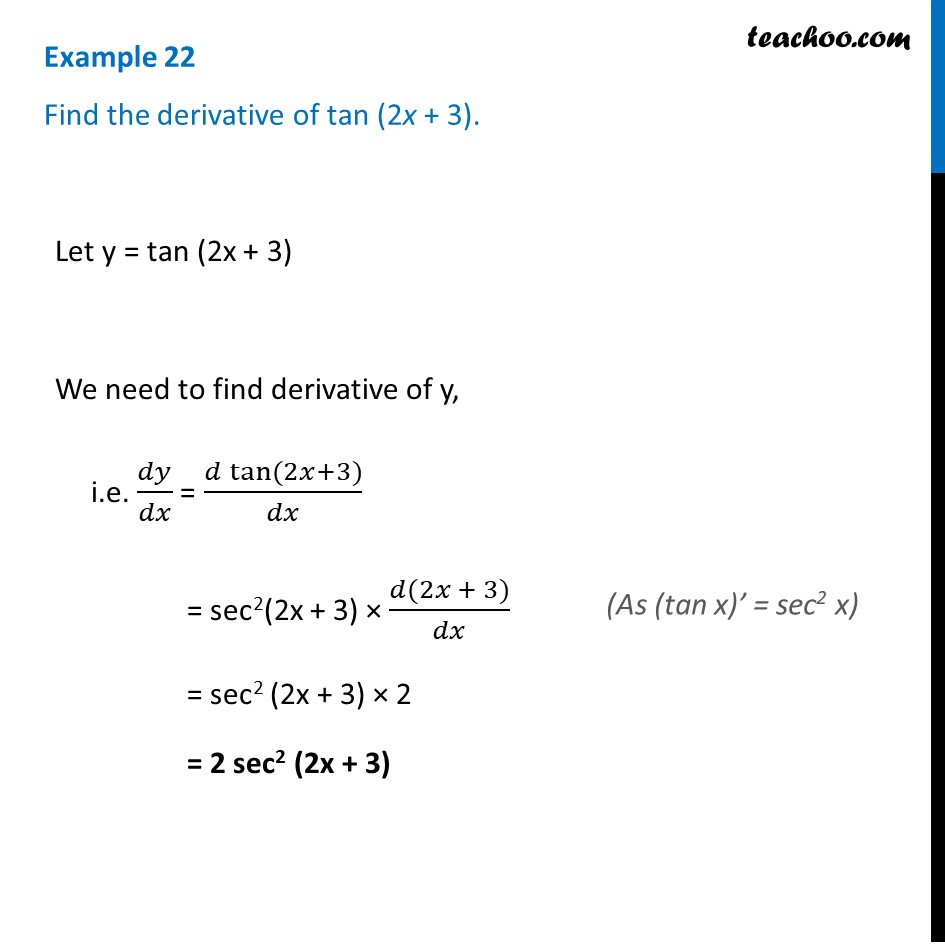



Example 22 Find Derivative Of Tan 2x 3 Chapter 5 Class 12



Tan2x ただの悪魔の画像




Differentiate The Following By Using First Principle I Tan 2x 1 Ii Tan X Iii Maths Limits And Derivatives Meritnation Com
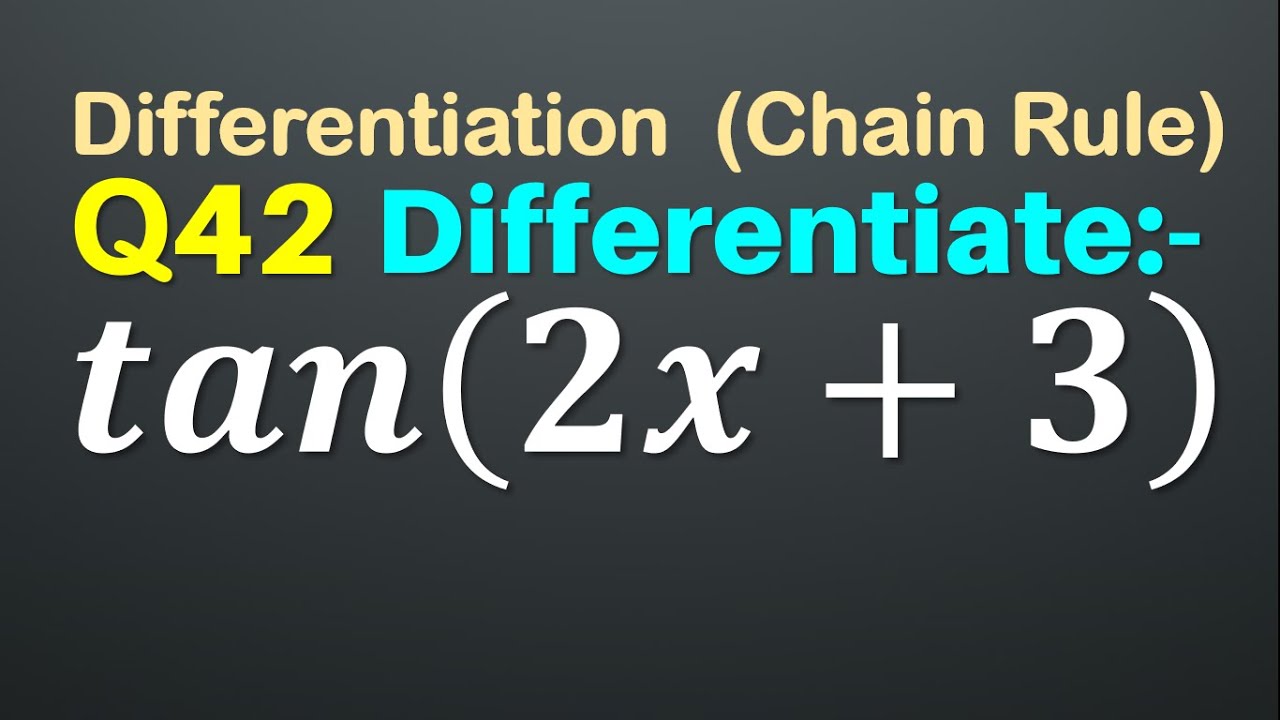



Q42 Differentiate Tan 2x 3 Derivative Of Tan 2x 3 Differentiation Of Tan 2x 3 Youtube
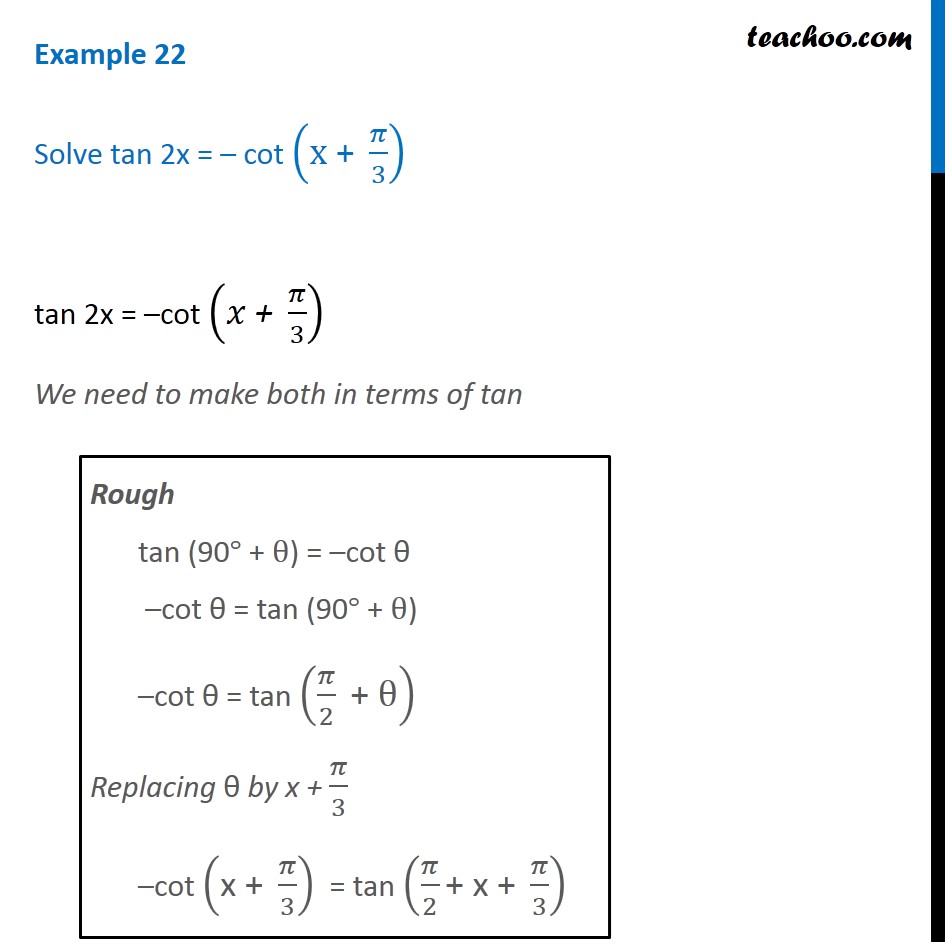



Example 22 Solve Tan 2x Cot X Pi 3 Teachoo Examples



What Is The Derivative Of Tan Root X By The First Principle Quora




Weierstrass Substitution Wikipedia



1




Prove That Tan 8x Tan 6x Tan 2x Tan 8x Tan 6x Tan 2x




The Derivative Of Sec 2 X With Respect To Tan 2 X Is




Differentiation Of Tanx 2 Youtube




Lesson 3 6 Implicit Differentiation Objectives Use Implicit



What Is The Differentiation Of Sec Inverse Tan 2x Quora




Differentiation Of Tan 2 X And X 3 X 4 Youtube




How Do You Evaluate The Integral 1 Tan X 3 Sec 2 X Dx Within The Range 0 Pi 4 Socratic




Solve Tan X Tan 2x Sqrt 3 Tan X Tan 2x Sqrt 3




The Derivative Of H X 2 Sec 2 X Tan X Product Rule Example Youtube




How To Take The Derivative Of Tan X Video Lesson Transcript Study Com




Find The Derivative Of Tan 2x 3 Brainly In
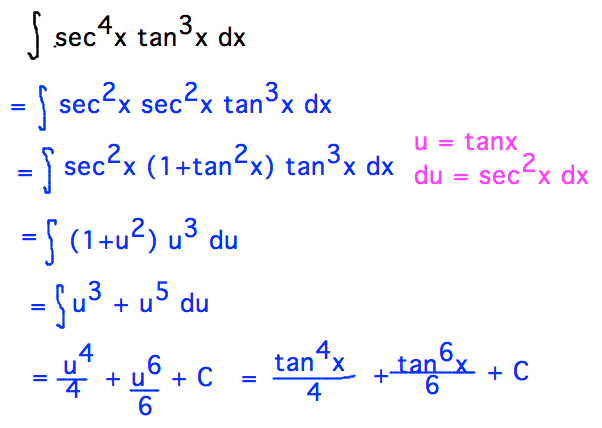



Geneseo Math 222 01 Trigonometric Integration



Early Geophysical Papers Of The Early Geophysical Papers Of The Society Of Exploration Geophysicists Earlygeophysical00soci Year 1947 Is Curvature Of Equipotential Surfaces 85 Dk D T R Sin 2x 2s



Find The Derivative Of The Function Tan 2x 3 From The Definition First Principles Sarthaks Econnect Largest Online Education Community
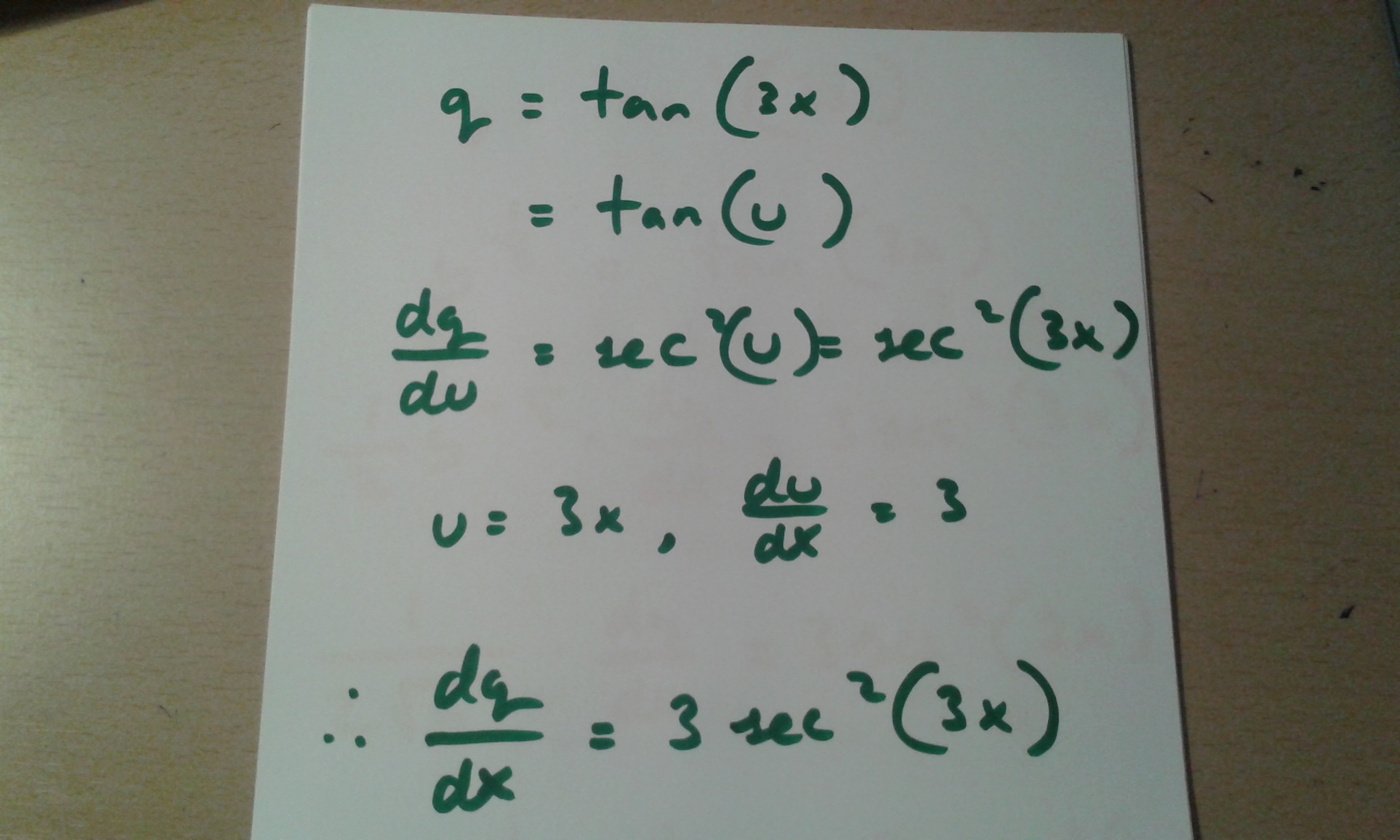



How Do You Find The Derivative Of Tan 2 3x Socratic




What Is The Derivative Of Tan 2x Socratic




Find Derivative Of Xsin2x 5 X K X Tan 2x 3 Q 13 X Sin 2x 5x Kx Tan2x3 Maths Limits And Derivatives Meritnation Com



Differentiate The Following Function With Respect To X X Sin 2x 5 X K K Tan 2x 3 Sarthaks Econnect Largest Online Education Community




Find The Derivative Of Tan 2x 3




Solved 10 Given That Y In Sec 2x 3 Find The Chegg Com




Differentiate Tanx Tan 2x Tan 3x Tan 4x W R T X Youtube
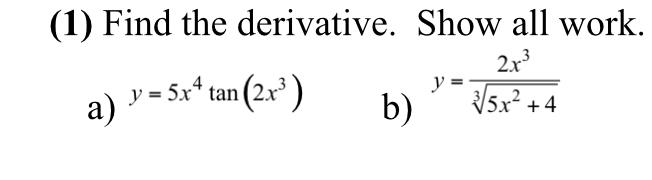



Solved 1 Find The Derivative Show All Work 2x3 A Y Chegg Com




How To Take The Derivative Of Tan X Video Lesson Transcript Study Com




How To Take The Derivative Of Tan X Video Lesson Transcript Study Com



Find The Derivative Of Tan 2x 3 Youtube




Show That Tan3xtan2xtanx Tan3x Tan2x Tanx Mathematics Topperlearning Com Dc9390xx
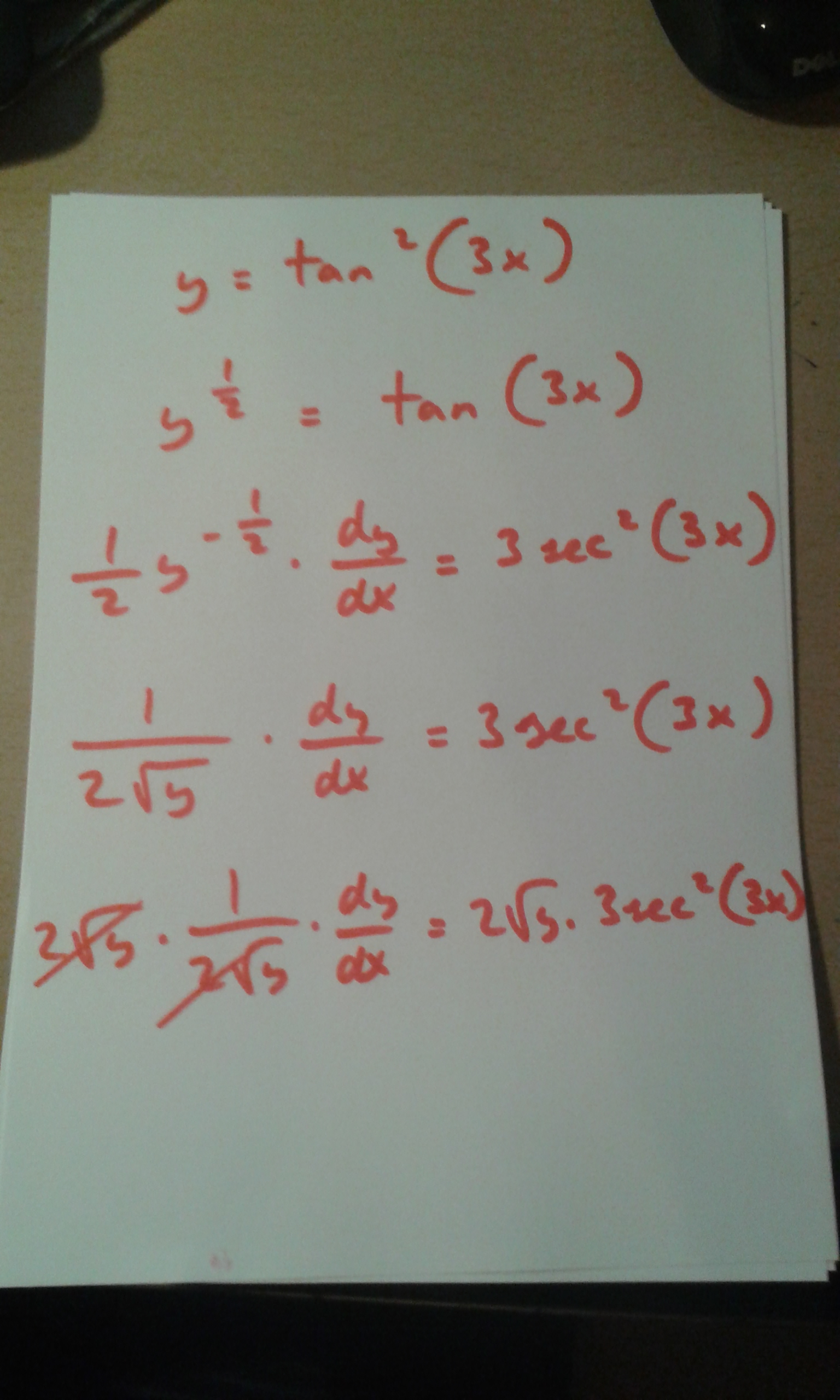



How Do You Find The Derivative Of Tan 2 3x Socratic




Derivative Of Tan X Old Video Khan Academy




Tan2 Derivative ただの悪魔の画像
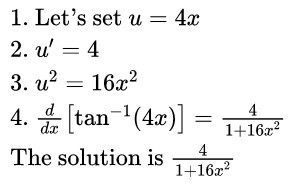



Derivative Of Arctan X Inverse Tangent Detailed Lesson




Find The Derivative Of Y Tan 3 2x Youtube
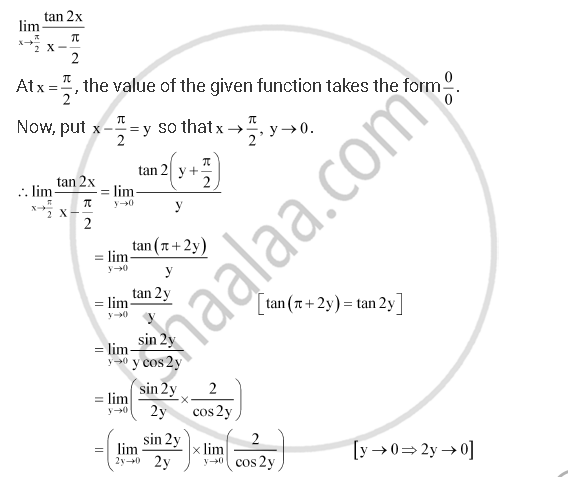



Evaluate The Given Limit Lim X Pi 2 Tan 2x X Pi 2 Mathematics Shaalaa Com




If Y Tanx Tan2x Tan3x Then Dy Dx



Tan2x ただの悪魔の画像



What Is The Nth Derivative Of Arctan 2x 1 X 2 In Terms Of R And Theta Quora




Solved Y F Then Find The Derivative Dy Dx 1 V V1 4x 3 Chegg Com
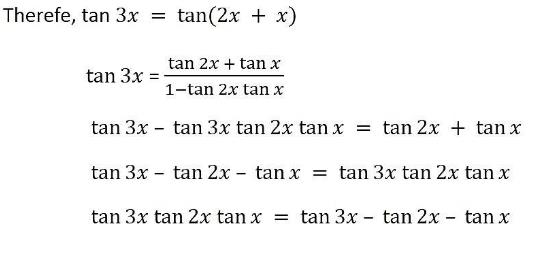



Prove That The Differentiation Of Tan3x Tan2x Tanx Is 3sec 3x 2sec 2x Sec X Edurev Class 12 Question
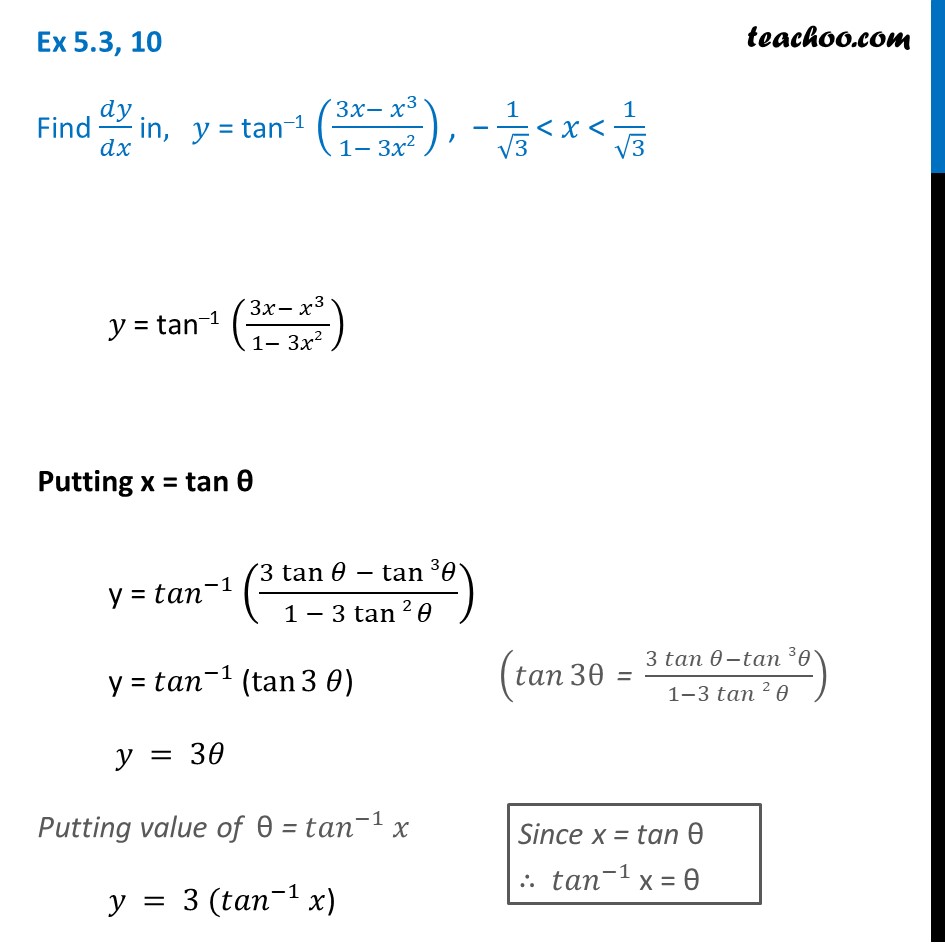



Ex 5 3 10 Find Dy Dx In Y Tan 1 3x X3 1 3x2 Ex 5 3




The Derivative Of Tan 2x W R T Cos 2x Is Maths Limits And Derivatives Meritnation Com




Weierstrass Substitution Wikipedia




Differentiate The Following From First Principle Tan2x Youtube




Solved Please Show All Steps And All Work 3 Find The Der




Find The Derivative Of The Given Function Y Tan 2x 1 Cot 2x I Tried Converting The Original Function In Terms Of Sin And Cos But It Was Still Too Complicated To Be Called Simplified




Prove That Derivative Of Tan X Is Sec 2 X By First Principle



最新 Tan2x Derivative ただの悪魔の画像




Ex 7 3 15 Integrate Tan3 2x Sec 2x Class 12 Ncert Ex 7 3




Derivative Of Tan 2x 3 From First Principle Brainly In




Solved Find The Derivative Show All Work 2x3 A Y 5x4 Chegg Com



Tan2x Sec2x ただの悪魔の画像
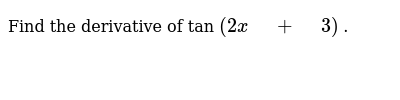



Find The Derivative Of Tan 2x 3




Y Tan 3 X Find The Derivative Youtube




Solved Find The First And The Second Order Partial Chegg Com




F Nd Derivative Of Tan 2 X 3




Tan2x ただの悪魔の画像
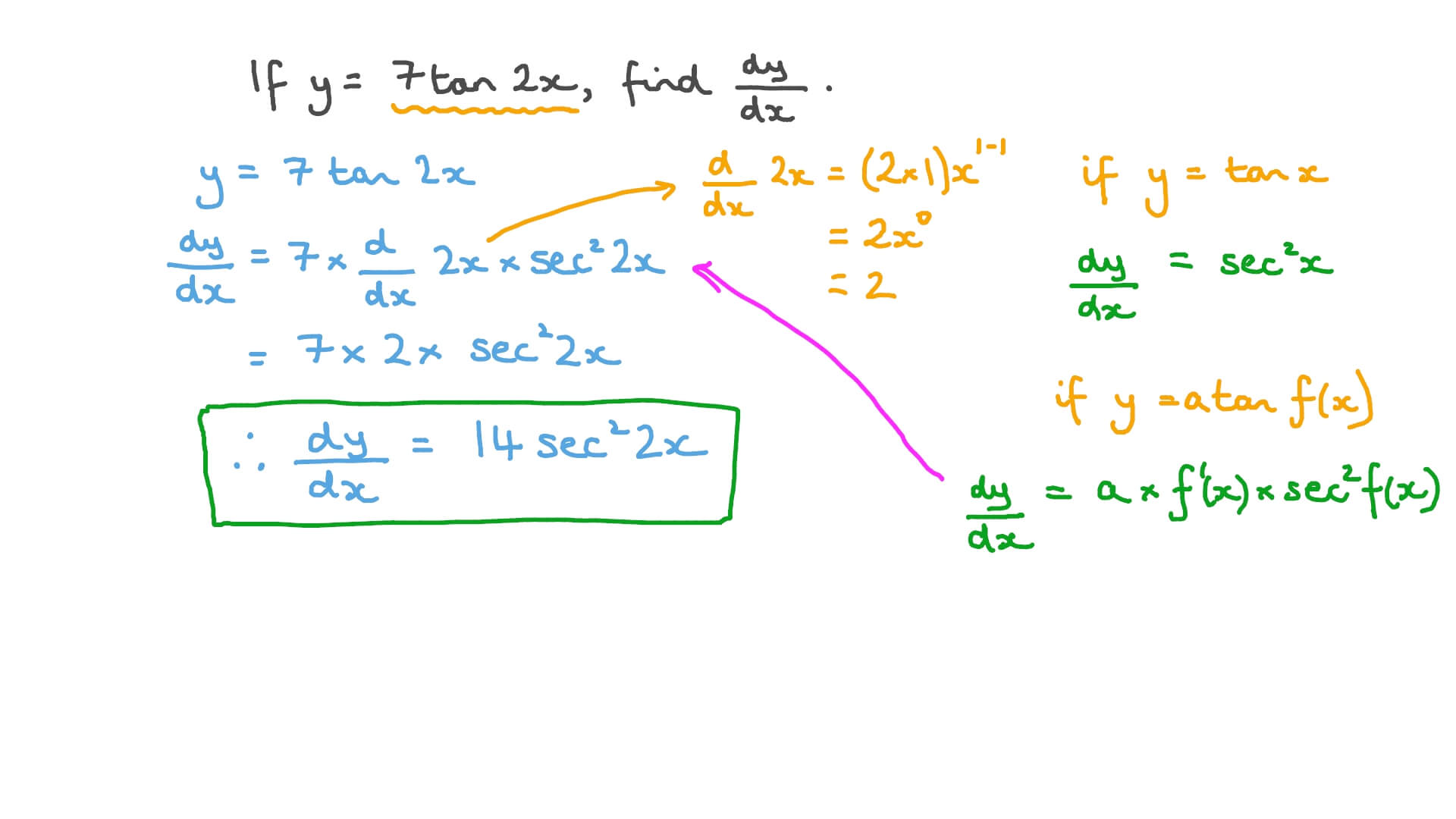



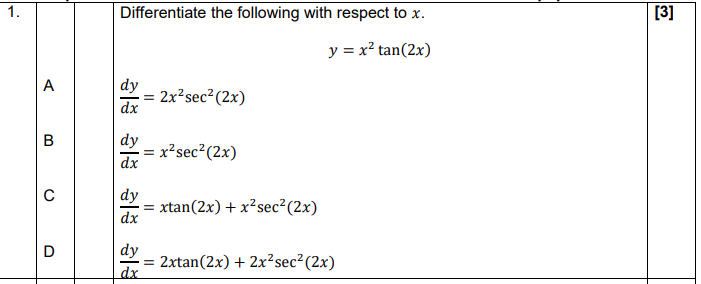
Question Video Differentiating Trigonometric Functions Nagwa




What Is The Integration Of Tan 2x Solution Quora




Find The Derivative Of Tan 2x From First Principle Brainly In
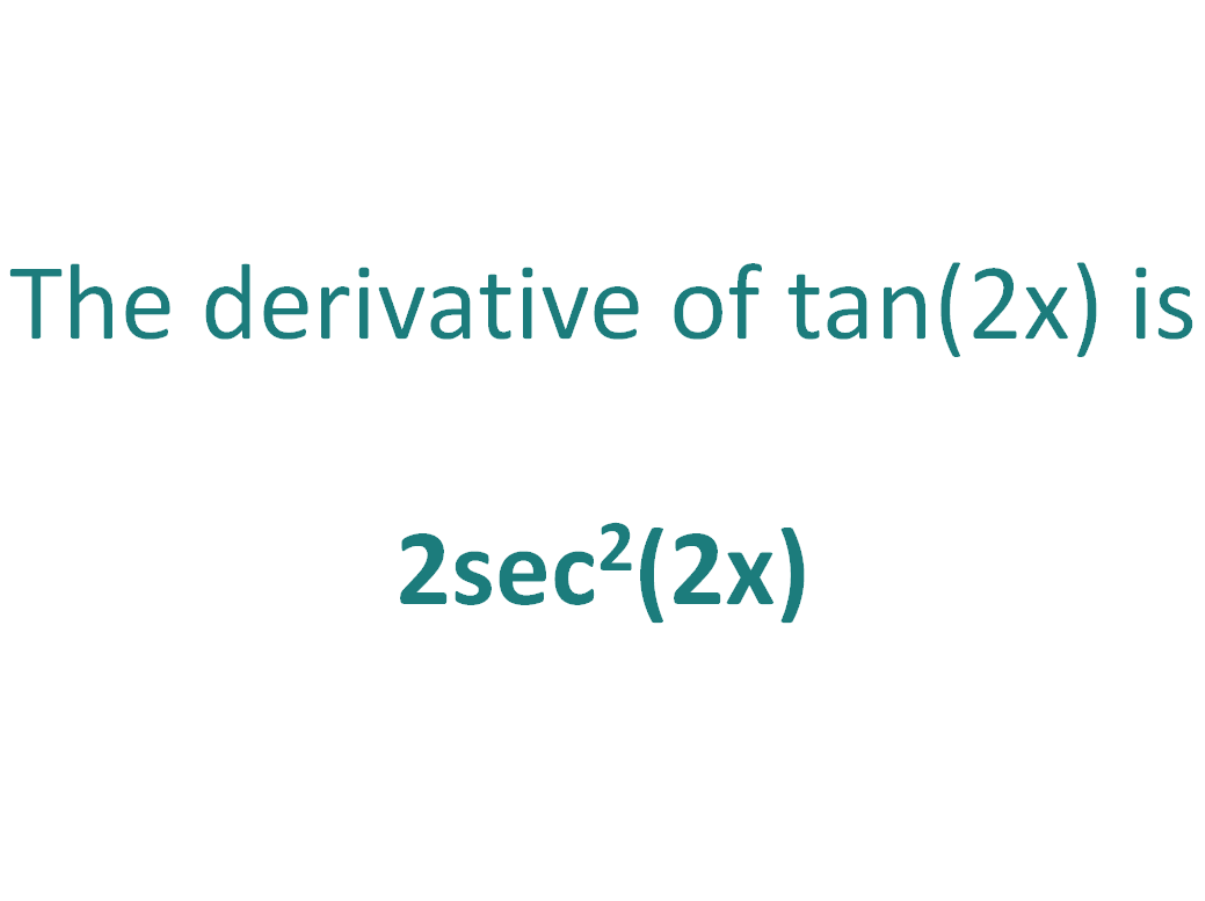



The Derivative Of Tan 2x Derivativeit


